We are happy to announce the preliminary agenda for the CAESES Users Meeting 2019! We’ll have leading companies with us, such as MTU, KSB, Suzlon, Aramco Services and Artemis Racing. See the event page for all details and registration.
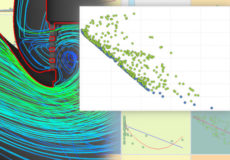
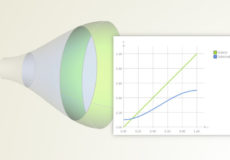
When we talk about CFD optimization software, we actually think of a an entirely automated engineering process that covers geometry generation, meshing, simulation and design post-processing. All of these single steps are automated to create a closed optimization loop where, finally, the optimal design candidate(s) emerge from the process, showing optimal results in terms of the product’s flow behavior (e.g. minimum pressure loss, lowest drag, reduced wave resistance, flow homogeneity, robustness for different operating conditions, etc.).
We have an updated version of the CAESES ACT app, to integrate CAESES into the ANSYS Workbench.
Learn the basic concept of meta surfaces in CAESES, to create advanced parametric sweep surfaces with more controls.
Not registered yet for the CAESES Users Meeting in Berlin? You can still benefit from the early bird rates until May 31, 2019. Almost 2/3 of the available seats have been taken already, so register today and make sure you will be part of this event! We also recommend to book your hotel room as soon as possible – Berlin will be crowded in September.
With CAESES version 4.4.2, we introduced a set of new features to automate the CFD software CONVERGE for the purpose of conducting design explorations and shape optimization. This short article gives you an update on the existing CAESES capabilities and their function in the context of using CONVERGE as your CFD software.
In this blog post, we’ll take a quick look into an automated workflow for the fast and flexible design of propeller CAD models that are also suited for automated shape optimization with CFD (Computational Fluid Dynamics).
It’s spring time over here in Europe and we are excited to announce that the new CAESES release is out now! Even though this is formally a maintenance release, we have added a few new capabilities based on user requests from the last couple of months. Check out the changes log for all details, and browse through the picks below for an overview. Thanks to everyone who provided feedback and input for CAESES 4.4.2!
“Can we analyze STL data, e.g. from a 3D scan, and extract 3D blade sections using CAESES?”. Well, this is a question we have heard several times in the last weeks and months. From a product point of view, if several users are asking for a specific functionality, there must be a substantial need for it. So, we started a more detailed discussion with them, to find out what they really want to achieve, and where they want to go in the end.
With CAESES 4.4.2, we’ll introduce a new surface type, the Gordon surface. This surface can be seen as a complementary tool for situations where you would create Coons patches in CAESES. So, what it does is, allowing you to create a surface patch based on a given network of curves. This set of U- and V-curves needs to be ordered for generating the parametric surface.